From Stress ➝ A*
in 3-6 Months
Real Students. Real Results.
Late nights. Endless past papers. Turn C into A* in 3-6 months. 500,000+ students did.
⚠️ Limited spots available
⚡ EXAM SEASON ALERT: Book Now or Risk Missing Your Dream University
25-50% Score Boost
Proven results in 3-6 months
Premium IGCSE, IB & A-level Tuition
Up to 70% off - Quality You Trust, Prices You'll Love!
AI Powered Resources
200K+ past papers, 5K+ notes, 1K+ videos
83% of our students scored As/A*s
Proven track record of excellence!
The Real Reasons You're Struggling
"My tutor legit didn't know the Cambridge mark scheme."
Most tutors aren't fully trained in the exact syllabus and marking schemes. You need someone who actually knows the game!
"I studied for weeks but still blanked in the exam."
Without targeted diagnostics, weaknesses remain hidden until it's too late. Our AI spots what you can't see!
"Ran out of time AGAIN."
Students lose marks due to inefficient answering techniques and poor time management. It's not just about knowing the answers, it's about playing smart!
Don't blame yourself. You don't need more hours — you need smarter hacks. That's where we come in.
Experience how we identify and fix your learning gaps
We're Your Exam Crew
Your complete solution for crushing exams without the stress. We've got your back with AI-powered matching and world-class tutors.
AI finds your perfect tutor in 2 minutes
Our AI analyzes 50+ factors to match you with the ideal tutor for your learning style and goals.
World-class tutors who actually know your syllabus
Cambridge & IB examiners, subject specialists, and award-winning teachers. No more tutors who don't get it!
AI Buddy: instant past paper feedback
On 100K+ real exam questions with auto-marking, gap analysis & custom study plans. Practice makes perfect!
Tools & strategies to crush exam anxiety
Stay calm under pressure with proven techniques and mental preparation strategies that actually work.
Proven 25-50% score boost in 3-6 months
Verified by thousands of students — Real results that you can see in your grades and confidence.
Start your journey to exam success with your perfect tutor match
From Struggling ➝ A* in 3-6 Months
Real students. Real photos. Real transformations. See how our method helped them achieve their goals.

Natasha Ho Zhi-Ying
"9 As at IGCSE. Honestly shocked myself.*"
Improved 3 Grade Levels to A* • IGCSE Co-ordinated Sciences

Atriya Dutta
"C ➝ A in 3-6 months. Not even kidding.*"
Improved 3 Grades to A* • All IGCSE Subjects

Ghassan Ammar
"Biology finally makes sense!"
Improved 5 Grade Levels • IGCSE Biology

Sunny
"Math is actually fun now!"
Improved 3 Grade Levels • IGCSE Math & Science

Aditya Sil
"Perfect IB score: 45/45. Feels unreal."
45/45 in IB Diploma • IB Maths

Alexandra Tanihana
"Chemistry clicked overnight!"
Improved 3 Grade Levels to A* • IGCSE Chemistry

Aditya Agarwal
"A* in all 9 subjects. No cap!"
A* in all 9 IGCSE Subjects • All IGCSE Subjects

Saudha Kitchilan
"English is now my superpower!"
Improved Grade Level to A* • IGCSE English & Accounting
87% of students book within 24 hours - Don't miss your spot!
⚠️ *Real student testimonials. Limited spots available.
Tutopiya's crew is small, elite & leveling up their grades every day.
📚 Top tutors ✔️ Exclusive tips ✔️ Winning community ✔️
Spots are limited… don't get left behind! 🔥
Top IGCSE, IB & A-Level Tutors Ready to Help You Excel
Connect with qualified tutors specializing in IGCSE, International A-Levels, and IB Diploma programmes. Each tutor is carefully selected for their expertise in your specific curriculum and subjects.
IGCSE Specialist Tutors
Expert IGCSE tutors covering all subjects from Mathematics to English Literature. Get personalized support for Cambridge and Edexcel curricula.
IB Diploma Mentors
Experienced IB tutors for all 6 subject groups including TOK, Extended Essay, and CAS guidance. Achieve your target grades with confidence.
A-Level Subject Experts
Cambridge and Edexcel A-Level specialists in Sciences, Mathematics, Humanities, and Languages. Master complex topics with expert guidance.
Curriculum-Specific Approach
Each tutor is trained in your specific exam board requirements. No generic teaching - just targeted exam preparation.
Featured IGCSE, IB & A-Level Tutors
Limited Slots Available!
Top tutors are booking up fast - secure your spot today
AI That Actually Gets You! 🤖✨
No more boring study sessions! Our AI is like having a super-smart study buddy who knows exactly what you need, when you need it. Get instant help, personalized feedback, and actually understand where you're crushing it and where you need to level up! 🚀
🎯 Smart Practice Tests That Actually Help
Stop guessing what you need to study! Our AI creates practice tests that are just like the real exams, then tells you exactly where you're slaying and where you need to put in work. No more wasting time on stuff you already know! 💪
🔮 Your Future Grade Predictor
See where you're headed before the exam!
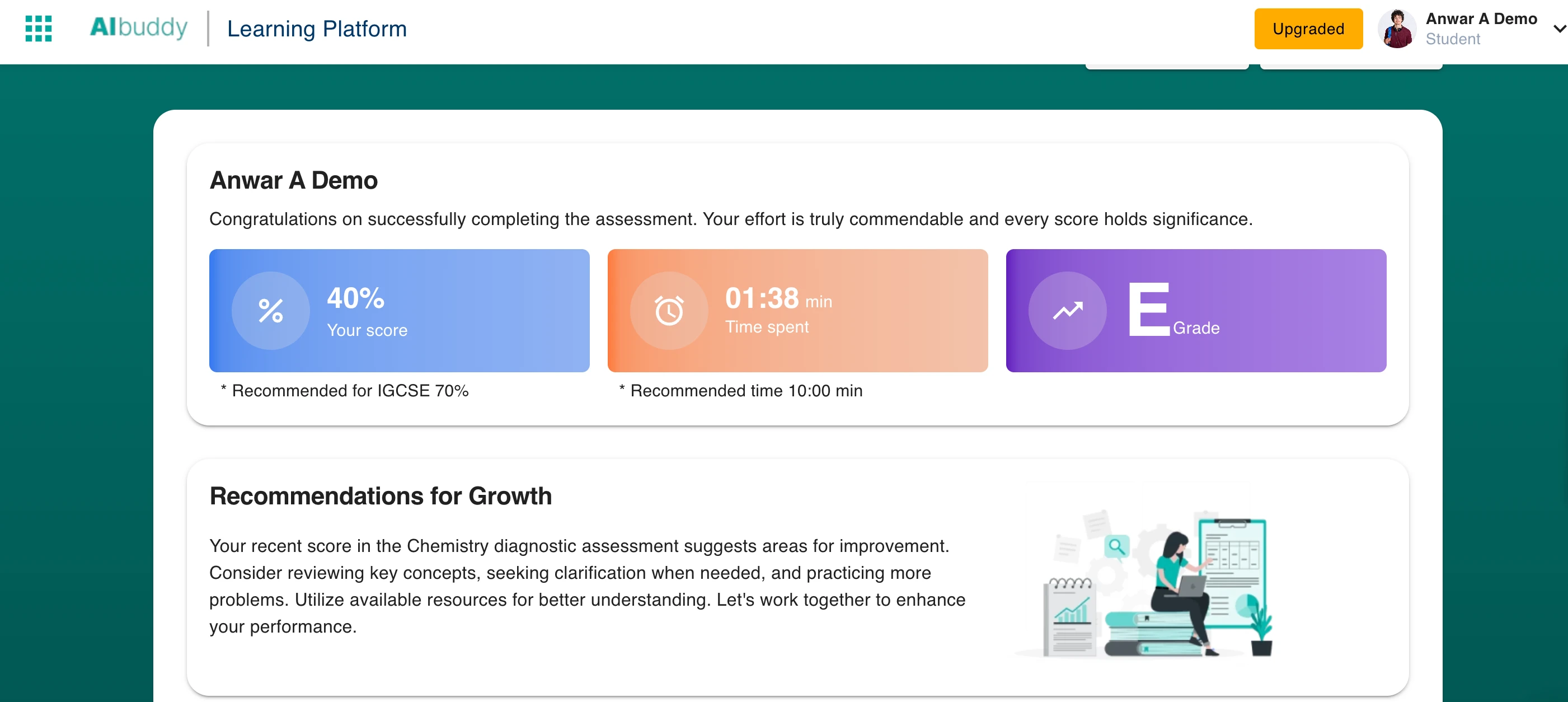
💪 Your Superpowers & Weak Spots
Know what you're crushing and what needs work!

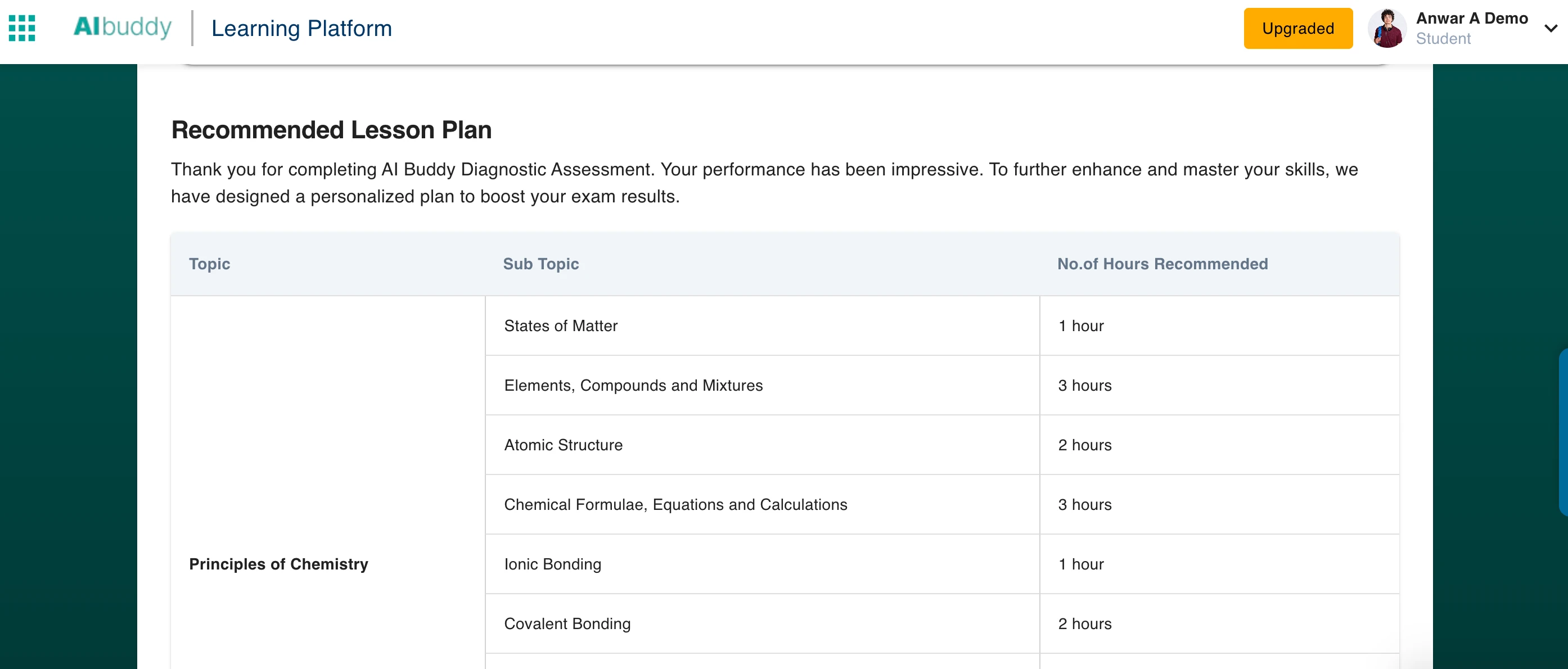
📚 Your Personal Study Roadmap
A custom plan made just for you!
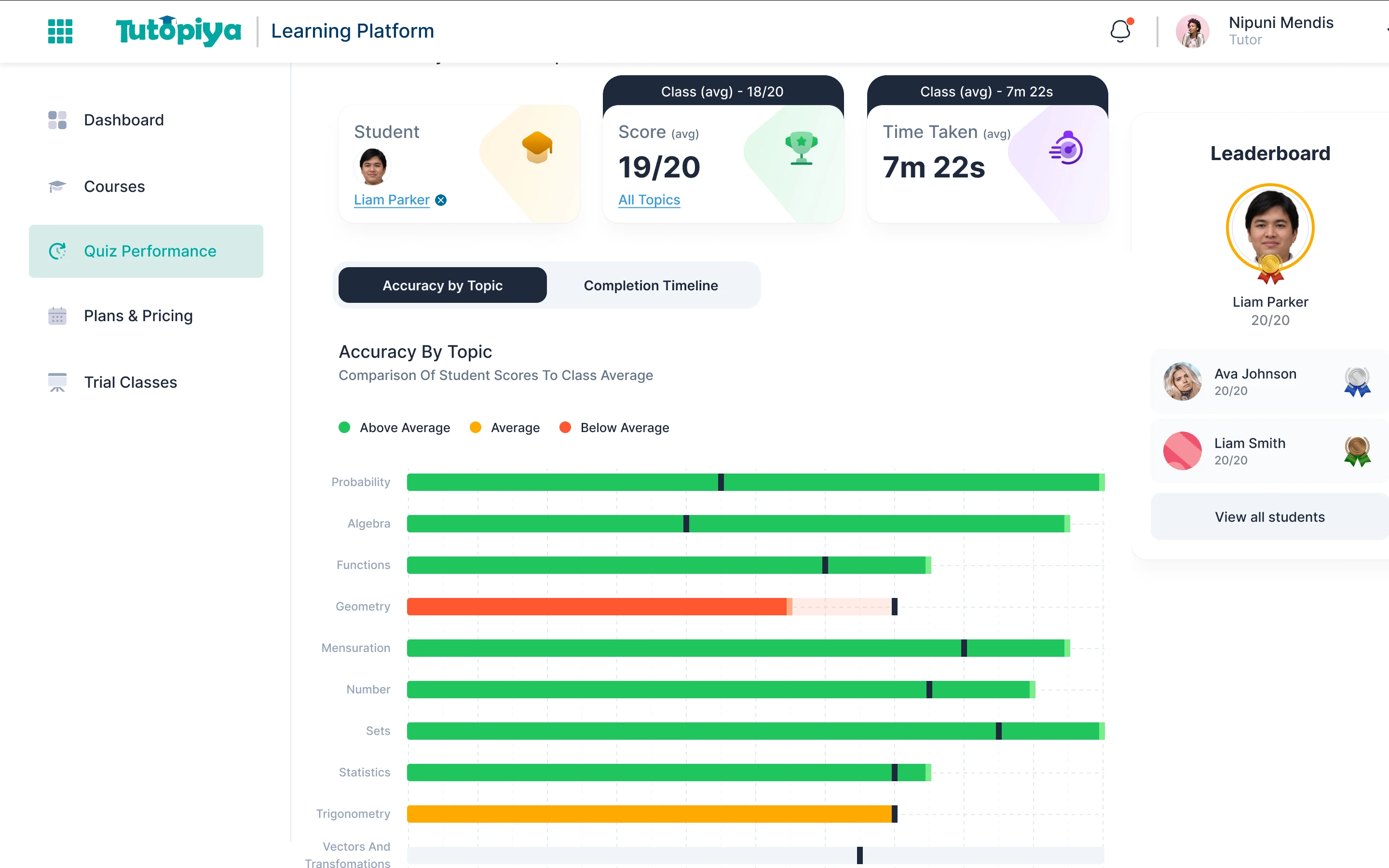
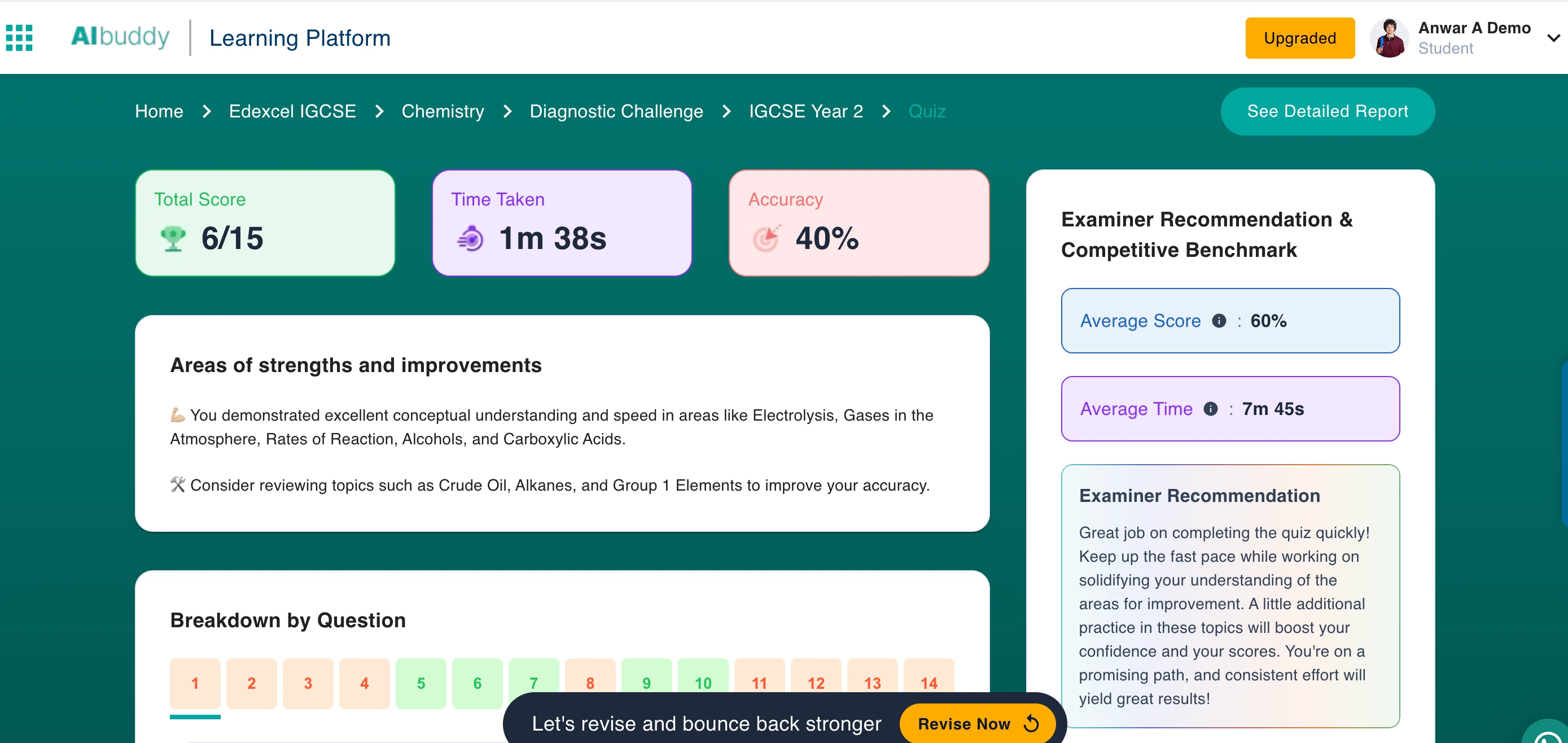
📊 Your Performance Dashboard
It's not just about getting the right answers - it's about getting them FAST! Our dashboard shows you exactly which topics are eating up your time so you can speed up and ace those exams! ⚡
🏆 Score Tracking
See how you're doing vs your classmates!
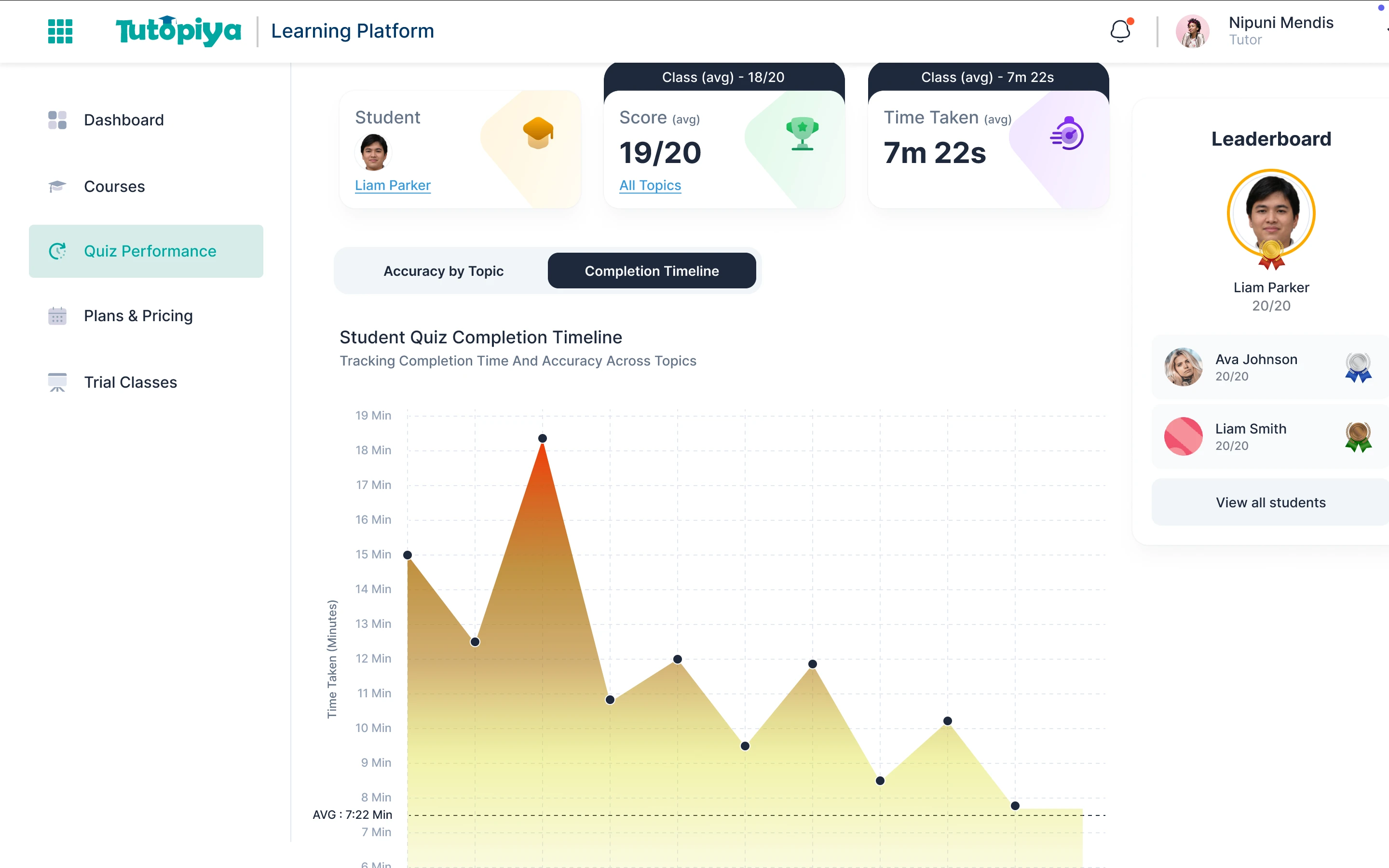
⏱️ Speed Tracker
Find out what's slowing you down!
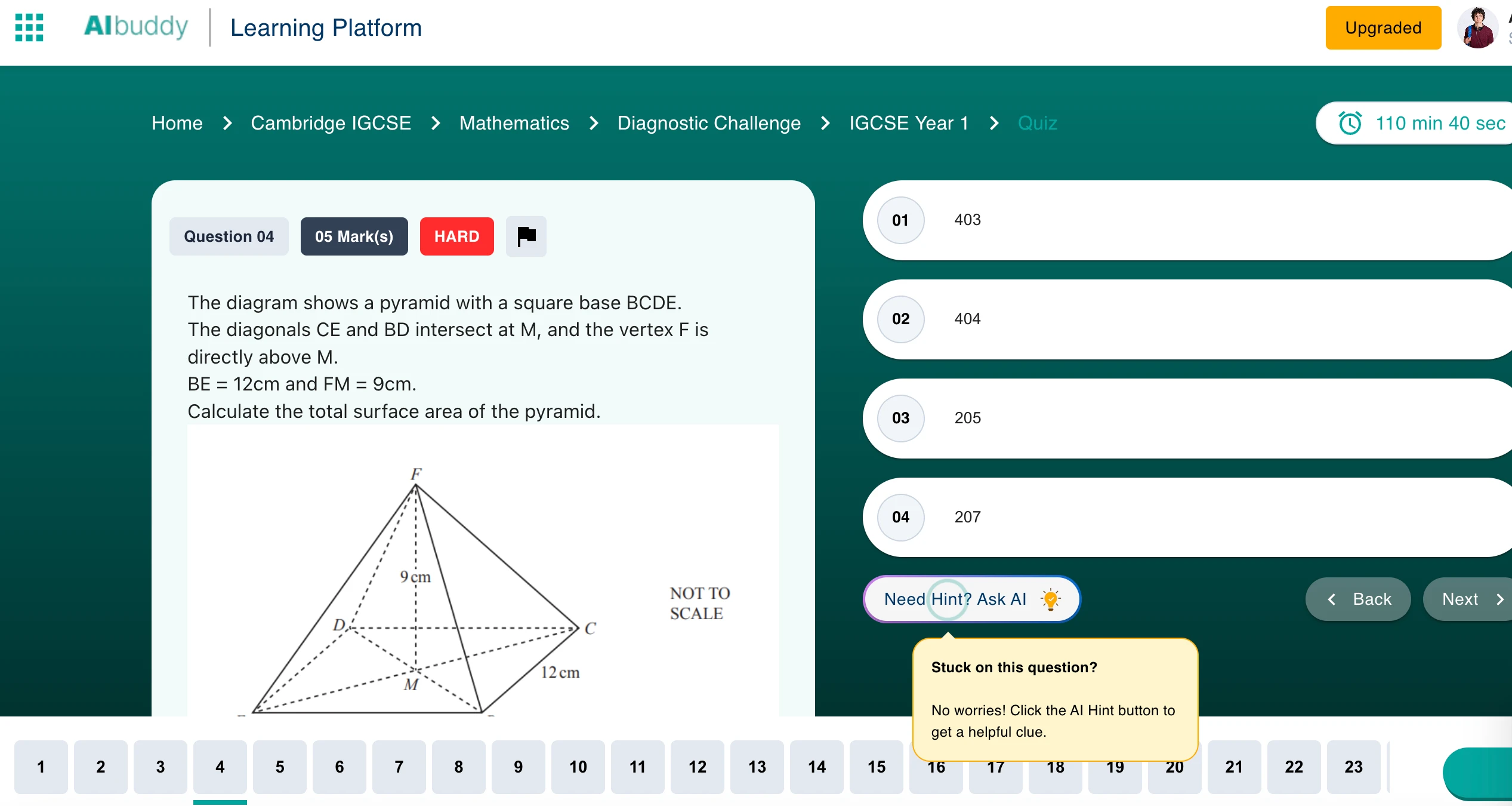
🎯 Your Personal Exam Coach
Imagine having a real examiner sitting next to you, telling you exactly what you did wrong and how to fix it! Our AI gives you instant feedback that's actually helpful - no more guessing why you lost marks! 💡

🤖 Your 24/7 Study Buddy
Stuck on a question? Don't panic! Our AI is like having a genius friend who's always there to give you the perfect hint - not the answer, but just enough to get your brain working! No more staring at blank pages! 🧠✨
💡 Smart Hints That Actually Help
Get unstuck without getting spoiled!
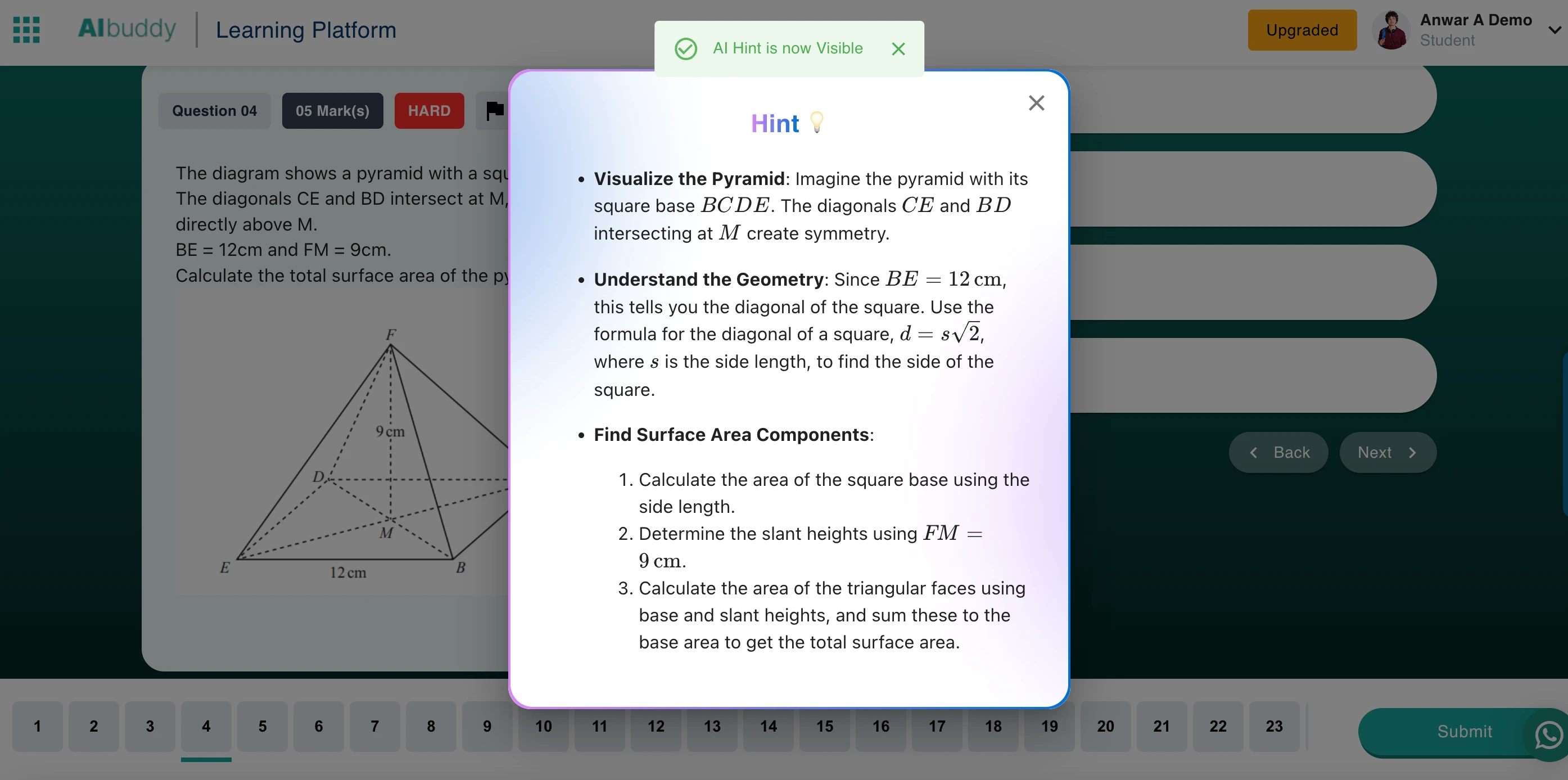
🧩 Step-by-Step Solutions
Learn the process, not just the answer!
🏆 See How You Stack Up Against Your Peers
Want to know if you're actually doing well? Our AI compares you with students just like you and gives you the real tea on where you stand! No more wondering if you're behind or ahead - you'll know exactly where you rank! 📊
📈 Track Your Progress Like a Pro
See how you're doing compared to your whole class! Our AI shows you exactly where you stand and helps you understand what you need to focus on to get ahead! 🎯

🏆 Awards & Accreditations
We're not just another tutoring platform - we're award-winning and officially recognized! Check out all the cool awards and certifications we've earned! 🎉
Award-winning platform
Join thousands of successful students!
Free IGCSE & A-level Resources - No Sign Up Required!
Access our comprehensive collection of free resources including study materials, practice papers, revision guides, video lessons, and more to ace your exams.
Comprehensive study materials for Cambridge IGCSE Extended curriculum with AI-powered practice and detailed video explanations.
Mathematics
(0580) - Extended
Comprehensive IGCSE Mathematics resources covering algebra, geometry, trigonometry, and statistics with AI-powered practice questions and video lessons.
Physics
(0625) - Extended
Master IGCSE Physics with interactive simulations, detailed video explanations, and thousands of practice questions with AI feedback.
Chemistry
(0620) - Extended
Comprehensive IGCSE Chemistry resources with virtual labs, molecular visualizations, and extensive practice materials.
Biology
(0610) - Extended
Explore IGCSE Biology with interactive diagrams, 3D models, and comprehensive study materials for all topics.
English Language
(0500) - Extended
Develop your IGCSE English Language skills with writing workshops, reading comprehension exercises, and speaking practice.
Computer Science
(0478) - Extended
Learn IGCSE Computer Science with programming tutorials, algorithm visualization, and practical coding exercises.
Complete study materials for Pearson Edexcel IGCSE curriculum with comprehensive topic coverage and exam preparation resources.
Mathematics
(4MA1) - Higher Tier
Edexcel IGCSE Mathematics resources with comprehensive coverage of higher tier topics and exam preparation.
Physics
(4PH1) - Higher Tier
Edexcel IGCSE Physics with practical experiments, theory explanations, and exam-focused practice materials.
Chemistry
(4CH1) - Higher Tier
Edexcel IGCSE Chemistry resources with comprehensive topic coverage and experimental techniques.
Advanced study materials for A-Level students with in-depth content coverage and university preparation resources.
Mathematics - Cambridge
Cambridge (9709)
Cambridge A-Level Mathematics with advanced calculus, complex numbers, vectors, and applied mathematics.
Physics - Cambridge
Cambridge (9702)
Cambridge A-Level Physics covering mechanics, electricity, waves, quantum physics, and practical skills.
Mathematics - Edexcel International
Edexcel International
Edexcel International A-Level Mathematics with pure math, mechanics, statistics, and decision mathematics.
All Resources Are Completely Free! 🎉
No hidden costs, no sign-up required. Start learning right away and transform your grades!
Trusted by Students from Leading International Schools Around the World
Join thousands of students from prestigious international schools who trust Tutopiya for their IGCSE and A-Level success.
Singapore
Leading International Schools
UAE
Premium International Education
Hong Kong
Excellence in International Education
Malaysia
World-Class International Schools
India
Premier International Education
Saudi Arabia
Excellence in International Education
United Kingdom
Home of IGCSE & A-Levels
Thailand
Premier International Education
Indonesia
Growing International Education
Why Students from Leading Schools Trust Tutopiya
Proven Results
25-50% score improvement in 3-6 months
Expert Tutors
Cambridge & Oxford graduates with 5+ years experience
Comprehensive Resources
200K+ questions, 1K+ videos, 10K+ study notes
Join Students from These Prestigious Schools
Experience the same high-quality education that students from leading international schools receive.
We're More Than Just Grades
We get it. School isn't just about exams — it's about confidence, growth, and your future.
Our Mission
Make world-class exam prep accessible, affordable, and anxiety-free for every student.
Your Goals
Whether you're aiming for top A*s, your dream uni, or just passing without panic — we're here to back you all the way.
We're On Your Team
Fighting stress, building confidence, and making sure no student feels left behind.
Real Talk
School is tough. Exams are stressful. But you don't have to face it alone. We've got your back.
Ready to Transform Your Academic Journey?
Join thousands of students who've found their confidence, improved their grades, and discovered their potential with Tutopiya.
🚀Start Your TransformationThe Media Can't Stop Talking About Us! 🔥
From major news outlets to radio stations — everyone's buzzing about how we're revolutionizing exam prep for Gen Z!











💸 High-Quality Tutoring, Low-Key Prices
No scam, just serious value! ✅
Why pay more when you can get the best for up to 70% less than traditional tuition centers?
Your payments are safe with us
Tutopiya's Payment Guarantee PolicyHere's the deal:
We hunt down the world's top tutors
Global marketplace means access to the best talent from around the world.
Curriculum & subject specialists
Experts who know your exact syllabus inside and out.
IGCSE, IB & A-level examiners
Actual examiners who know exactly what the markers are looking for.
Top International School teachers
Educators from the world's leading international schools.
Heads of Departments
Senior educators with years of leadership experience.
How We Keep Prices Low:
We negotiate smart rates thanks to our scale and bring you tutors from markets where living costs (and rates) are lower—so you get the best of both worlds.
And yes, we've got your back:
Nothing but the best for your grades—and your wallet.
Start with a FREE trial lesson and see the difference quality tutoring makes.
🔥Book Your Free TrialStop Stressing. Start Winning.
Your perfect tutor is waiting. Your exam success is within reach. What are you waiting for?
No commitment. No pressure. Just results.
Join 10,000+ students who've already transformed their exam performance
🎉 Join the Tutopiya Crew – Limited Spots, Unlimited Smarts
Not everyone can get in… but you can. 😎
Tutopiya isn't just tutoring—it's a club of top students leveling up their grades with the world's best tutors.
Hang out (virtually!) with other motivated teens
Connect with students who are just as driven as you are. No more studying alone!
Get exclusive tips, hacks & exam strategies
Insider knowledge that only Tutopiya students get access to. The secret sauce to success!
Be part of the group that's crushing IGCSE, IB & A-levels
Join the elite community of students who are already achieving their dreams. Winners only!
Limited spots = elite, high-achieving community
We keep our community small and focused. Quality over quantity!
⏰ Don't miss out—everyone's leveling up, are you in or out?
Spots fill up fast. Join the students who are already transforming their grades and future.
"I was skeptical at first, but now I'm part of the crew that's actually getting A*s!" - Sarah, IGCSE Student
Join 2,847+ students who've already leveled up their academic game
📱 Tutopiya = Learning Made Crazy Easy
We've spent 7 years building the ultimate study setup—so you can focus on smashing exams, not tech headaches.
Mobile app (iOS & Android)
Learn anywhere, anytime. Your study buddy in your pocket!
One-click class join
No stress, just click & go. Super simple!
5,000+ tutors to pick from
Find your perfect match from our global network. The choice is yours!
200,000+ auto-marked questions
Real past paper questions with instant feedback. Practice makes perfect!
1,000+ video tutorials
Learn at your own pace with expert explanations. Visual learning made easy!
5,000+ revision notes
Comprehensive study materials for every topic. Everything you need!
Secure chat support
Ask questions anytime, get help instantly. Never stuck again!
All lessons recorded
Replay & review anytime you need. Never miss a thing!
Smart analytics
Spot gaps & create personal study plans. AI-powered insights!
AI instant feedback & hints on past papers
Get personalized help, instant explanations, and smart hints that actually make sense.
Study smarter, not harder—Tutopiya's got you covered.
🚀Start Learning Smarter TodayJoin thousands of students who've already discovered the easier way to study
IGCSE, IB & A-Level Tuition That Actually Works
Whether you're crushing IGCSE exams, surviving the IB Diploma, or conquering A-Levels - we've got your back with expert tutors who know these curriculums inside out.
IGCSE Tuition
Your shortcut to A* grades in IGCSE subjects
📚 Popular IGCSE Resources:
A-Level Tuition
Master A-Levels for your dream university
🎯 Popular A-Level Resources:
Free IGCSE & A-Level Study Tools
Access our comprehensive collection of free study tools including mock exams with certificates, the largest IGCSE student forum, past paper finder, and more to ace your exams.
Join the Student Community
Connect with fellow students, get study tips, and find motivation in our supportive community. You're not alone in this exam journey!
Study Forums & Communities
Connect with students worldwide
Latest Study Tips & Insights
Get the latest exam strategies and study hacks from our expert tutors
Got Questions?
We've got answers! Here are the most common questions students and parents ask about our tuition services.
🤔How quickly can I see results with Tutopiya?
Most students see a 25-50% improvement in just 3-6 months. Our proven method combines expert tutoring with AI-powered resources to accelerate your learning. Some students even see results in as little as 3 months!
💰How much does Tutopiya cost compared to traditional tuition?
We're up to 70% cheaper than traditional tuition centers while providing premium quality. You get expert tutors, unlimited access to 200K+ past papers, and AI-powered study tools - all for less than what most centers charge for basic tutoring.
👨🏫Are your tutors qualified for IGCSE, IB & A-levels?
Absolutely! All our tutors are certified experts with extensive experience in Cambridge IGCSE, IB Diploma, and A-level curriculums. They've helped thousands of students achieve A* grades and get into top universities worldwide.
⚡How fast can I start learning with Tutopiya?
You can start learning in just 2 minutes! Our AI-powered platform matches you with the perfect tutor instantly, and you get immediate access to all our study resources. No waiting, no delays - just pure learning!
📚What subjects do you cover for IGCSE, IB & A-levels?
We cover all major subjects including Mathematics, Physics, Chemistry, Biology, English Language & Literature, Business Studies, Economics, and Computer Science. From Cambridge to Edexcel to IB - we've got you covered!
🌍Do you teach students from different countries?
Yes! We teach students from over 20 countries globally. Our platform is designed for international students, and our tutors understand the unique challenges of different education systems and time zones.
🎯Can Tutopiya help me get into my dream university?
Absolutely! Our students regularly get into top universities like Oxford, Cambridge, Harvard, MIT, and more. We don't just help with grades - we provide university application guidance and interview preparation too.
🔄What if I'm not satisfied with the tutoring?
We offer a 100% satisfaction guarantee. If you're not happy with your tutor, we'll match you with a new one immediately. Your success is our priority, and we're committed to finding the perfect tutor for you.
Still Have Questions?
Our expert team is here to help! Get personalized answers to all your questions about IGCSE, IB, and A-level tuition.
Global Tuition & Tutoring for International Students
Whether you call it "tuition" or "tutoring" - we provide world-class online education support for students across Asia, Europe, and the Middle East.
Singapore
Home Tuition & Online Classes
Malaysia
International School Support
UAE
Expatriate Education Support
United Kingdom
Online Tutoring Excellence
India
International Curriculum Support
Global
Worldwide Online Support
Popular Search Terms We Cover
Frequently Asked Questions
What subjects do you offer tutoring for?
We offer expert tutoring for IGCSE, A-Level, and IB subjects including Mathematics, Physics, Chemistry, Biology, Economics, English, and more. Our tutors specialize in all major international curricula.
How quickly can I see improvement in my grades?
Most students see significant improvement within 3-6 months, with an average 25-50% score boost. Our proven methodology and expert tutors ensure consistent progress.
Are your tutors qualified and experienced?
Yes, all our tutors are highly qualified with extensive experience in their subjects. They undergo rigorous screening and training to ensure the highest quality of education.
How does the free trial work?
We offer a free trial lesson where you can experience our teaching methodology, meet your potential tutor, and see how our platform works before committing to regular sessions.











