The article title is Introduction to Exponential Growth and Decay [Please watch the video attached at the end of this blog for a visual explanation of exponential growth and decay]   In this article we will be talking about exponential growth and decay; terms that sound like they are taken out of a Physics or Chemistry lesson and not Mathematics, but are actually a part of the topic of “Numbers” as well!   (You can access other articles on this topic here!)   What is Exponential Growth? Exponential means an extremely rapid increase (ex: size or extent). Therefore, exponential growth is when a quantity increases by the same factor over equal intervals of time, very rapidly.   Examples: Population growth The population in most countries increases very rapidly. Bacterial growth Viruses Viruses usually multiply within seconds of entering a host body. This is also exponential growth.  We use a formula to get the value of exponential growth. A = P (1 + r/100) to the power n In this,  A = final amount  P = principal amount  n = number of years   To arrive at the final answer A, the principal amount P is multiplied by a particular ratio that we invest in, to the power of a number of years (n).   What is Exponential Decay? Exponential decay, if we rely on the meaning of exponential, means a decrease in a quantity of something by the same factor over equal intervals of time.   Exponential decay is therefore a decrease from the original amount by a percentage each year (r%) for n number of years, and it can be measured using the formula below. A = P (1 - r/100) to the power n The only difference you will see between this formula and the formula for exponential growth is that this formula has a subtraction sign (-) whereas the exponential growth formula has an addition (+) sign.    Therefore, another way to differentiate the two formulas is to have different signs.   Examples:  Water cooling down  The value of a car decreases over time Radioactive decay If you consider the example question given below, we first list down the information provided. We list the information as P = 17,000, r = 25, n = 8. 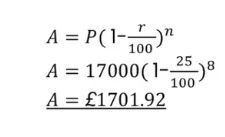We then substitute all the values into the formula, and then you get the answer as shown below: Let’s make revision easier! Pay close attention to the question. Are they focusing on exponential growth, decay, both or neither? Depending on the question, you have to change the formula that you use, so be mindful of what the examiner is asking!   Past paper questions tend to be simple, but students often make mistakes when substituting the values into the formula. This is why practice is important. Some questions can be found here as well, and you can time your answers to see if you can stick to the time limit given.   If you are struggling with IGCSE revision or Mathematics in particular, you can reach out to us at Tutopiya to join revision sessions or find yourself the right tutor for you.   Watch the video below for a visual explanation of exponential growth and decay. Make sure to attempt the quiz once you’re done! https://www.youtube.com/watch?v=GlghT2EI4qs